December 2, 2019

The European Renaissance was obsessed with classical antiquity. For many of its intellectuals it marked a cultural and scientific golden age. Many classical authors, among them the likes of Lucretius and Cicero, were rediscovered and celebrated. And among the many subjects given a new lease of life during the Renaissance was geometry. A branch of mathematics developed most significantly in ancient Greece, geometry was most famously formalized by Euclid in the fourth century BC. His Elements would be the primary text on geometry for the next 2,000 years.

Geometria
Today’s featured 5-minute book is not by Euclid or one of the other esteemed ancient authors, but was written by a much later and lesser-known German artist and mathematician, Augustin Hirschvogel (1503–53). His slim 100-page treatise, Geometria, was published in Nuremberg in 1543.* Hirschvogel was best known for his landscape etchings and sketches, but in this little book about geometry he applies his talents as an artist to illustrate numerous geometrical figures and diagrams. Among them are the famed five Platonic solids and methods for their construction.

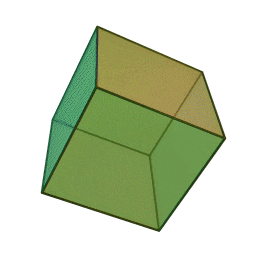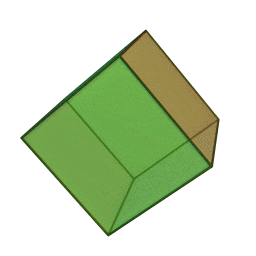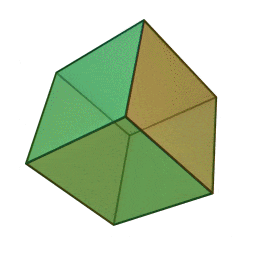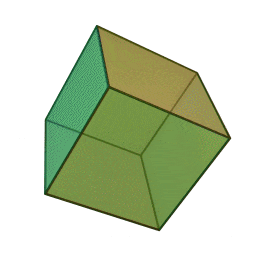
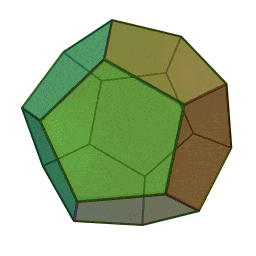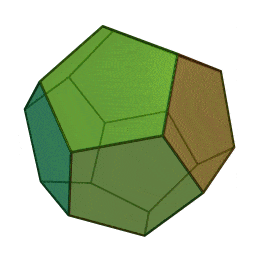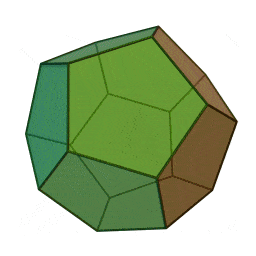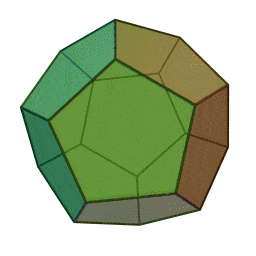
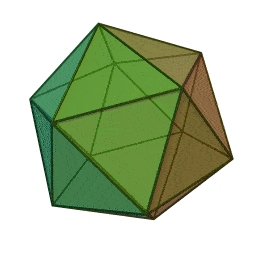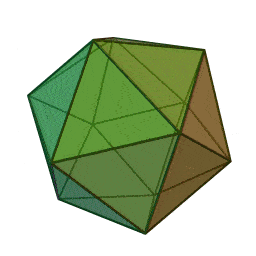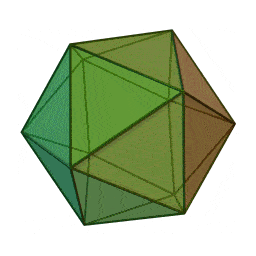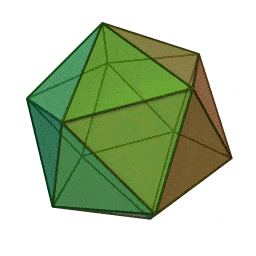
Although not the first to write about them, Plato was the first to clearly define what we now call the five Platonic solids (tetrahedron, cube, octahedron, dodecahedron, and icosahedron). Avoiding technical jargon, a Platonic solid is an enclosed 3-D object with identically shaped faces and vertices; for example, a cube, the second Platonic solid, has six identical square faces, and its eight corners or vertices are likewise identical. It’s pretty incredible when you think about it: that there are only five convex solids which meet these criteria.

The history of the five Platonic solids is a fascinating one. There isn’t space here, but I will just say that from very early on (before Plato) they were associated with the four fundamental building blocks or elements of the cosmos: water, earth, fire, and air. Even the brilliant Renaissance astronomer Johannes Kepler, in his Mysterium Cosmographicum (1596), attempted to explain the distances and orbits of the five extraterrestrial planets with relation to the proportions of the five Platonic solids, a quite beautiful, albeit fatally flawed conception.

And if the universe were governed by geometry, then surely even art at its best would be based on geometric principles. Among the many Renaissance artists fascinated by geometry was Paolo Uccello (1397–1475); so much so that the sixteenth-century art historian Giorgio Vasari wrote that Uccello seemed more a mathematician than an artist. For the brilliant Renaissance architect and artist Leon Battista Alberti, art was a descriptive language governed by geometry. He was the first to codify the principles for geometric drawing in his 1435 treatise, De pictura (‘On Painting’).

During the fifteenth century most images were printed from woodcuts. They were relatively inexpensive and could be printed in the same forme as the metal type (both are relief printing methods). However, by the beginning of the sixteenth century, we begin to witness many more metal engravings. And, although they had to be printed on a separate roller press, they did permit finer detail and, with etching, made possible printing various tones. To save having to run the sheets through the press again to print the text separately, often ⁠— and this is so in the Hirschvogel etchings above ⁠— the text is engraved along with the images. ◉